A 0.450-kg hockey puck, moving east with a speed of 5.80 m/s, has a head-on collision with a 0.900-kg puck initially at rest. Assuming a perfectly elastic collision, what will be the speed and direction of each puck after the collision?

VIDEO TRANSCRIPT

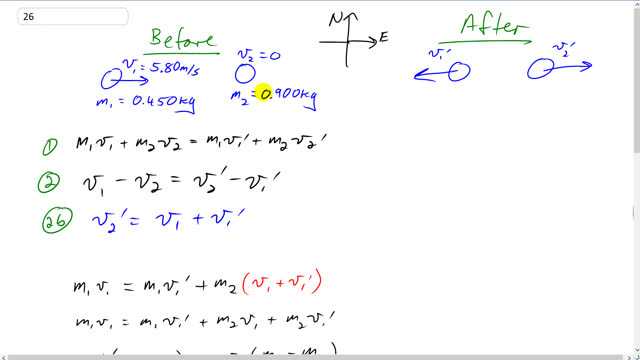
This is Giancoli Answers with Mr. Dychko. Before the collision of these hockey pucks, the first one is approaching at 5.80 meters per second as a mass of 0.450 kilograms and the second one is at rest with a mass of 0.900 kilograms and after they collide, they are both gonna be moving with some velocities probably although we don't strictly know what this one is gonna be doing; we can say for sure that this second puck is gonna be moving to the right to some extent but this first puck could either be moving to the right, it could stop and it could move to the left those are all possibilities. But I happen to know that when you have a collision like this where it's elastic and where one of them is initially at rest that this first incoming object— its velocity is given by this formula— it will come to a stop if its mass is equal to the second mass because, you know, this difference will be zero in which case, v 1 prime will be zero; mass 1 will move to the left if its mass is less than mass 2. So if a thing, that is initially at rest, is more massive then it will make the first thing rebound backwards which is the case here because this initially at rest thing here is 0.9 versus 0.450 kilograms for the thing approaching and there you go. So I just kinda know that the picture is gonna look like this. Now if you didn't know that that's fine because it's all gonna work out in the algebra and with the calculations because you are gonna end up with a negative answer for v 1 prime and so that negative sign tells you that it's moving to the left after the collision. So we'll start by writing down conservation of momentum and also write down this formula which we know because the collision is elastic and kinetic energy is conserved as kinetic energy. And so we can rearrange equation 2 by first getting rid of this term entirely because v 2 is zero— this object starts at rest— and we'll solve for v 2 prime by moving this term to the left side, adding it to both sides and then switch the sides around so we have v 2 prime is v 1 plus v 1 prime and the reason that's useful is that we can get rid of this v 2 prime variable in formula number 1 and we can also get rid of this term because v 2 is zero. So here's equation 1 written again where we have m 1v 1 equals m 1v 1prime plus m 2 and then never mind this v 2 prime, we can write v 1 plus v 1 prime there instead and so now we have a formula with only one unknown, v 1 prime and so we'll do some algebra to solve for it. We distribute this m 2 into the brackets by multiplying both terms by it so we have m 1v 1 equals m 1v 1 prime plus m 2v 1 plus m 2v 1 prime and these two terms have a common factor, v 1 prime, and so we'll factor that out and we end up with v 1 prime times m 1 plus m 2 and then this term goes to the other side so it becomes negative or subtracted from both sides, you could say, and you have m 1v 1 minus m 2v 1 and then v 1 is a common factor between these two terms. And so I have also switched the sides around so that we have the unknown v 1 prime on the left so we have that v 1 prime times m 1 plus m 2 on the left side now because I switched the sides around and we have v 1 factored out of this m 1 and then minus m 2 on the right side now and then divide both sides by m 1 plus m 2 and we have this formula here. And substitute in some numbers, we have v 1 prime then is 5.80 meters per second— velocity of the hockey puck when it's approaching before collision— times 0.450 kilograms minus 0.900 kilograms divided by the sum of the masses and this gives negative 1.93 meters per second. Now since it specifies that it's moving east, let's describe our directions with the words 'east' or 'west' instead of with a negative sign although using a negative sign is perfectly correct especially since we have defined our coordinate system there— I mean I guess I can go one step further and say, put a little plus sign there but that's kind of implied by the arrow pointing in that direction that that's the positive direction— and then we plug in that answer into this formula, v 2 prime is v 1 plus v 1 prime to figure out v 2 prime. So there we go and we are gonna substitute in a negative number there because the word 'east' or the word 'west' isn't something we can put into a formula. So we have 5.80 meters per second plus negative 1.93 and that gives 3.87 meters per second and since it's positive, it is 'east'.