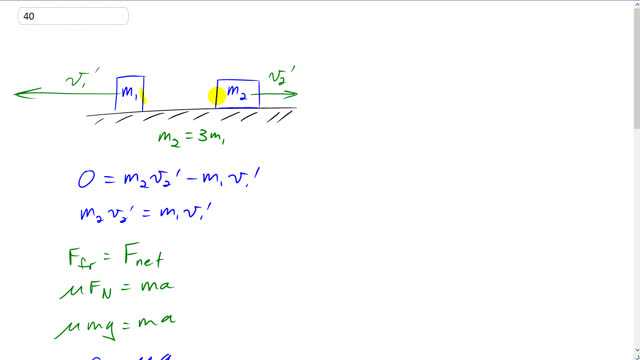
A wooden block is cut into two pieces, one with three times the mass of the other. A depression is made in both faces of the cut, so that a firecracker can be placed in it with the block reassembled. The reassembled block is set on a rough-surfaced table, and the fuse is lit. When the firecracker explodes inside, the two blocks separate and slide apart. What is the ratio of distances each block travels?

VIDEO TRANSCRIPT

This is Giancoli Answers with Mr. Dychko. This block has separated into two pieces; block two is 3 times the mass of block one and they are reassembled and the fire cracker is put in between and then the fire cracker explodes and separates them and they launch off with different speeds because they have different masses. The heavier one's gonna be at a smaller speed than the light one, m 1, it's gonna have a faster speed and therefore a greater distance traveled. So when we talk about conservation of momentum, initially there's no momentum in the system because they are attached together and at rest and then after the explosion, there's gonna be m 2v 2 prime to the right minus m 1v 1 prime to the left and that's why there's a minus sign there because that's gonna be negative velocity and that means m 2v 2 prime equals m 1v 1 prime. And that's as far as we can go when we talk about momentum, now we have to talk about force and kinematics in order to create an expression for distance and then we'll substitute what we just learned from momentum into that distance formula to sort things out. So the friction force is the net force on each of these blocks and friction force is μF N and F N is gonna be mg because there's no vertical acceleration so the normal force upwards applied by the table is gonna equal the force of gravity downwards on the block and that's gonna be ma and the m's cancel which means that the acceleration of each block is gonna have magnitude μ—coefficient of friction—times g— acceleration due to gravity. And then based on that, we'll plug that into this kinematics formula to figure out the distance that each block travels. So final velocity squared equals initial velocity squared plus 2 times acceleration times displacement and so displacement is gonna be negative v initial squared over 2a because each block comes to a rest— there's no final velocity— and then we'll take this to the left side and then divide both sides by 2a and a is μg as we just figured out here; I suppose I should write that substitution in red, there. And we don't care about displacement, we care about distance so we'll just take the absolute value of the displacement never mind the negative sign and so the distance each block travels will be the block's initial velocity squared divided by 2μg. So the distance block one travels divided by the distance block two travels which is the ratio of the distances— that's what we have to find here— is gonna be v 1 prime squared over 2μg and then multiplied by the reciprocal of the other distance— never mind dividing a fraction by a fraction, let's multiply by the reciprocal instead— and the 2μg cancels and the ratio of distances is the square of the ratio of their speeds. So we know how the speeds are related by conservation of momentum up here and that means that v 1 prime is m 2v 2 prime over m 1 and then we'll plug that in for v 1 prime here So we have m 2v 2 prime over m 1 all squared and then divided by v 2 prime squared but let's multiply by the reciprocal of v 2 prime squared so we'll multiply by 1 over v 2 prime squared and then these v 2 prime squared's cancel and we are left with the ratio of distance is the square of the reciprocal ratio of their masses. So d 1 over d 2 is m 2 over m 1 squared and m 2 is 3 times the mass of m 1 and so that's 3m 1 squared over m 1 squared; m 1 squared's cancel and we are left with 9. So the lighter block will travel 9 times the distance of the heavier block.