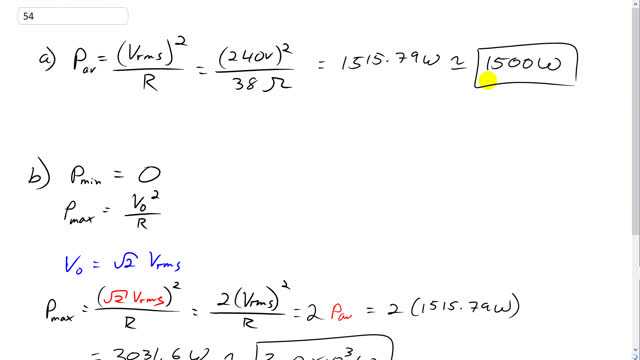
A heater coil connected to a $240$-$\textrm{V}_\textrm{rms}$ ac line has a resistance of $38 \; \Omega$.

VIDEO TRANSCRIPT

This is Giancoli Answers with Mr. Dychko. The average power dissipated by this heater coil is the R.M.S voltage squared divided by the resistance. So, that's 240 volts squared divided by 38 ohms which is about 1500 watts and the minimum power dissipated will be zero because as this voltage is oscillating at some point that'll be… it'll be zero. This is a zero point and here's the peak V naught and then there is negative V naught when it gets to the… you know, maximum at the negative side. This, you know, so it graph here, vertical axis is voltage and at some point it'll have zero voltage as it passes this horizontal axis here. And so the minimum power will be zero when there's zero voltage, there will be zero current and so on. Now the max voltage will be the peak voltage squared divided by resistance. This is the max power dissipated will be peak voltage squared divided by resistance. And so we can solve for V naught squared by multiplying both sides by… Well actually what we're going to do is we're going to substitute this root two V rms in place of V naught. That's what we're gonna do here. So peak voltage is root two times R.M.S voltage because we know R.M.S voltage and, and well actually R.M.S voltage squared over R is the average power which we've already calculated. So let's substitute that in. So we have root two times V rms, all squared divided by R is the same as two, because root two squared makes two to times V rms over R. and R.M.S squared over R is average power and so we have two times the average power is the peak power and that's going to be 3.0 times ten to the three watts.