A sphere of radius r = 34.5 cm and mass m = 1.80 kg starts from rest and rolls without slipping down a $30.0 ^\circ$ incline that is 10.0 m long.

VIDEO TRANSCRIPT

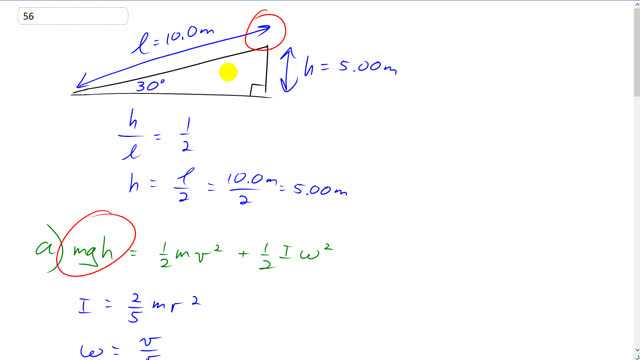
This is Giancoli Answers with Mr. Dychko. The total energy of this sphere when it's at the top of this incline is potential energy equal to mass times acceleration due to gravity, g, times its height above the reference level which we'll take to be the bottom of the incline. And so its this h that we are really interested in. We are given the length of the ramp which is 10 meters along the hypotenuse here and the angle of incline is 30 degrees and this is a special triangle where you have the opposite 1, hypotenuse 2, and this leg here root 3: 30 degrees, 60 degrees. And so without a calculator, we could see that this h over l is 1 over 2 and then multiply both sides by l and you get h is l over 2 so that's 10 meters over 2 which is 5 or you could... you could go sin 30 and figure it out that way knowing that sin 30 is h over l, you could multiply both sides by l here and see that h equals lsin 30 and you will notice that sin 30 is 0.5. Okay so total gravitational potential energy at the top since there's no kinetic energy there equals the total energy at the bottom— of which none is potential and it's all kinetic energy— we have translational kinetic energy and rotational kinetic energy. We'll substitute for the moment of inertia and angular velocity here. Moment of inertia for a sphere is two-fifths times it mass times its radius squared Rotational velocity is its translational velocity over r and it's useful to do this because then we have eliminated this ω variable and expressed it in terms of v and something that we know, r, and then we are gonna solve this for v in a second. So we have mgh equals one-half mv squared copied from here and then plus one-half times a substitution for the moment of inertia, two-fifths mr squared and a substitution for ω, v over r and that gets squared. So the r squared's cancel here the m is a common factor in all terms so we'll divide every term by m and we end up with, the 2's cancel here as well, so we have v squared over 5 from this right-hand term and then v squared over 2 there and then combine these two terms with a common denominator of 10 by multiplying this by 5 over 5 and this by 2 over 2 and you get 5v squared plus 2v squared over 10 equals gh; 5 and 2 make 7 over 10 v squared equals gh switching the sides around then multiply both sides by 10 over 7 and then take the square root of both sides and you get v is square root 10gh over 7. And we can see from this that the translational velocity at the bottom of the ramp does not depend on the radius of the sphere nor on the mass. So translational velocity is 10 times 9.8 times 5 meters and then divide it by 7 and square root that whole thing and you get 8.37 meters per second. The angular velocity is the translational velocity divided by the radius so we have 1 over r times square root 10gh over 7. So that's 1 over 34.5 centimeters converted into meters by multiplying by 1 meter per 100 centimeters which turns it into 0.345 and so I took this answer here divided by the radius in meters and you get 24.3 radians per second. The ratio of translational kinetic energy to rotational kinetic energy is one-half mv squared over one-half moment of inertia times ω squared. The one-halves cancel and substituting for I for a sphere and angular velocity there, the m's cancel and the r squared's cancel here these v squared's cancel and so we have 1 divided by two-fifths which is the same as 1 multiplied by its reciprocal of the denominator 1 over 5 over 2 and so the answer is 5 over 2. And so translational kinetic energy is a lot more than rotational kinetic energy, 2.5 times more. So only angular velocity depends on radius and nothing depends on the mass.