Two identical $+9.5 \; \mu \textrm{C}$ point charges are initially 5.3 cm from each other. If they are released at the same instant from rest, how fast will each be moving when they are very far away from each other? Assume they have identical masses of 1.0 mg.

VIDEO TRANSCRIPT

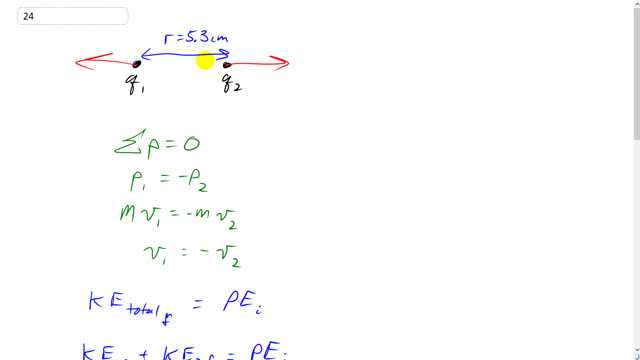
This is Giancoli Answers with Mr. Dychko. We have to bring in a little bit of conservation of momentum to this situation. The momentum initially, since they both are at rest, at 5.3 centimeters apart, the initial total momentum of the system is zero. And since there's no external forces acting on the system, the final momentum, after we release them, will still be zero. And, I mean there are internal forces to this system, but that doesn't affect the total momentum. So, the momentum that this charge one has to the left, is gonna equal the momentum this charge two has to the right: equal in magnitude, but it'll be in the opposite direction, so one is the negative of the other. So mv one equals negative mv two. And, both have the same mass, which are both one milligram. And so, v one is gonna be negative v two. Okay. So that's gonna be useful on this next train of thought, where we say that the total final kinetic energy of the system, will equal the initial potential energy that it has. So, all of the potential energy that it initially has turns into kinetic energy, 'cause we wait until they get really far away from each other, to the point where the final potential energy is zero. So, this total kinetic energy final, is the kinetic energy of particle one, plus the kinetic energy of particle two. And that's gonna be two times the kinetic energy of one of them, because they'll have the same magnitude of their velocity, same speeds in other words and they have the same masses, so we can say two times the kinetic energy of one of them is equal to the potential energy that they have initially. So, the initial potential energy of the system is one charge multiplied by the potential due to the other charge. So that's Q times k Q over r. And that's k Q squared over r. And that equals two times the kinetic energy of one of them, one-half mv f squared, and the two's cancel, and you can divide both sides by m, and then square root both sides, and you get these. Final speed is k Q squared over m r, square rooted. So that's square root of Coulomb's constant, 8.988 times 10 to the nine Newtons meter squared per Coulomb squared, times 9.5 times 10 to the minus six Coulombs, in each charge, squared divided by 5.3 times 10 to the minus two meters, that's their initial separation, times one-point o times 10 to the minus 6 kilograms. And don't get tricked up by this milligrams business. This is milligrams is times 10 to the minus 3 grams, but grams itself is times 10 to the minus 3 kilograms. So, these two times 10 to the minus three is getting multiplied together. So, a total of 10 to the minus six. 3900 meters per second is the final speed for each particle.