VIDEO TRANSCRIPT

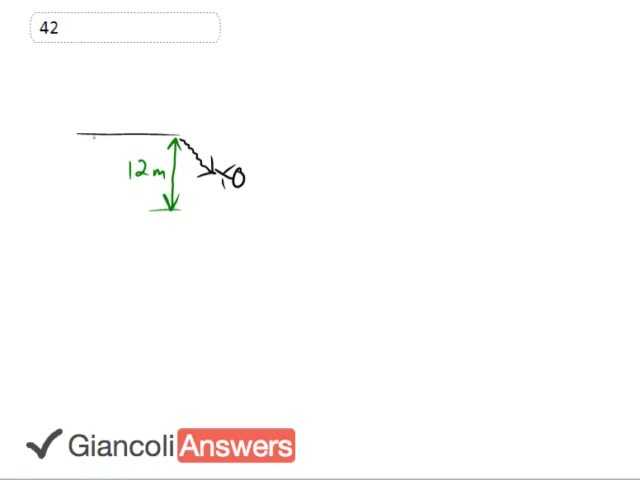
So this bungee jumper falls 12 meters before the bungee cord actually engages. So, it's slack until 12 meters. And the bungee jumper falls a total of 31 meters from the bridge. And then they stop at that point and spring back up. So, initially with these distances here, this is the stretch in the bungee cord, so that's 'x' is 31 minus 12 meters, which is 19 meters. So we're going to be calculating 'k', what is the spring constant of the bungee cord? Such that all this happens. And we'll start by saying that the initial potential in kinetic, add up to the final potential in kinetic. We know that initially there is no kinetic energy, so this term will be 0. And at the bottom of this, the bungee cord is fully stretched right at the bottom here; there'll be no kinetic energy. So that simplifies things a bit. So we have this 'mgh', where 'h' is measured with respect to this very bottom point here. 'Mgh' equals one half ''kx' squared. So the total gravitational potential that the bungee jumper started with at the top of the bridge, equals the total elastic potential they have, when the bungee cord is fully stretched. So in part a, we're going to solve this for 'k', and so we'll divide both sides by 'x' squared, and multiply both sides by 2. This gives us that 'k' is 2 'mgh' over 'x' squared and we'll substitute in numbers. That's 2 times 62 Kilograms; times 9.8 meters per second squared, times 31 meters, divided by 19 meters squared, then 19 meters, this is amount that it was stretched. And in the 5th edition by the way, this is 60 Kilograms, I've just mentioned that now, because it's the only change, and it actually doesn’t even change the answer. You get 104 Newtons per meter, which works out to 1.0 times 10 to the 2 Newtons per meter. And that's, this final answer rounded to 2 sig. figs is the same for both the 5th and the 6th edition.

Part b we have to find the acceleration. What is the maximum acceleration? Well we know that in this stretch from the top of the bridge to this point where the bungee cord is no longer slack, the acceleration there is 9.8 because they were just free falling. 9.8 meters per second squared down. And then once they pass this position here, then they will be gradually experiencing more and more elastic force upwards. The more the bungee cord gets stretched, the more strongly it pulls the jumper upwards. And that will continue until we get to the very bottom position here. That's when the bungee cord is stretched the most. So that's where they're gong to experience the most force upwards, and they'll spring back up. This bottom position is where the greatest acceleration is going to occur. So we know that the net force, the force upwards due to the cord, minus gravity which is downwards, equals mass times acceleration. Solving for a gives us, 'kx' where I've substituted 'kx' in place of force in the cord, minus mg all divided by 'm'. And that is 104.35 Newtons per meter, from part a, times 19 meters of stretch, minus 62 Kilograms, times 9.8, divided by 62 Kilograms. And the answer is 22 meters per second squared, is the maximum acceleration. And in the 5th edition this is 60, and so is this, but it doesn't make enough difference; you'll get the same answer to 2 sig. figs.