VIDEO TRANSCRIPT

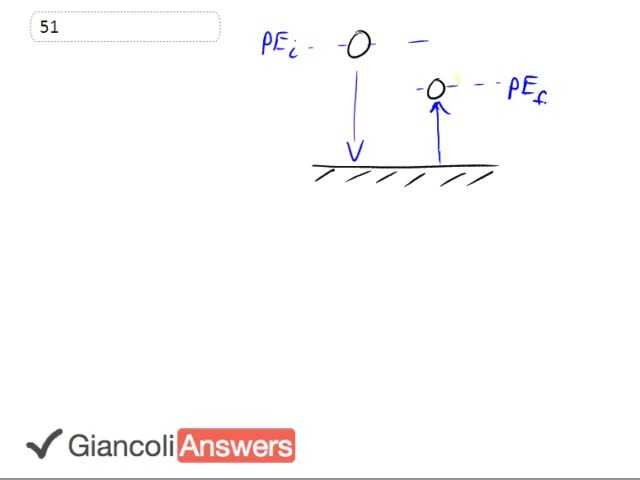
This ball starts at this initial position here and bounces up to it’s final position and the question is what is the percent… or the energy loss is what fraction of the initial energy? So we have the potential energy final minus the potential energy initial. That’s the amount that was lost divided by the… what it started with. So we have ‘mgh’ final minus ‘mgh’ initial divided by ‘mgh’ initial. And all these 'mg’s' are going to cancel then we’re left with something that looks a little better which is ‘h’ final minus ‘h’ initial divided by ‘h’ initial is the fraction of energy lost. So if 1.5 meters is the final height minus the 2.0 original height divided by 2.0. So that’s a loss of 0.25 or a one quarter loss of the initial energy. In part B the lift off speed must have happened here. So this is the kinetic energy at the ground. What kinetic energy did it have just as it was lifting off in order to achieve this height and this position? So we’re assuming there’s no friction due to air, but certainly there’s friction in the ground and in the particles of the ball itself. But we’ll talk about that in part C. So we have the kinetic energy on the ground equals the potential energy at the top of it’s path. It’ll have no kinetic energy there when it’s at the top. And we have one half ‘m’ velocity at the ground squared is 'mg' it’s final height. This gives us a speed from the ground [and] we’ll cancel the common factor ‘m’, multiply everything by 2 and take the square root of both sides. That gives the speed is the square root of 2 ‘ghf’. Square root of 2 times 9.8 meters per second squared times 1.5 meters. This gives a lift off speed from the ground of 5.4 meters per second.

In part C there… the energy that was lost was due to heat mostly; a little bit of sound energy must have been created as well, but mostly heat and when you’re playing squash for example, or some sport where you’re whacking a ball really hard, particularly squash, you can really feel the ball heating up and that’s due to friction among the particles inside the ball itself. So when the ball’s squished the ball particles rubbing up against it’s own ball particles is creating heat and there… be due to friction there and that’s where this energy is going, mostly heat.