A car is driven 225 km west and then 98 km southwest ($45^\circ$). What is the displacement of the car from the point of origin (magnitude and direction)? Draw a diagram.

VIDEO TRANSCRIPT

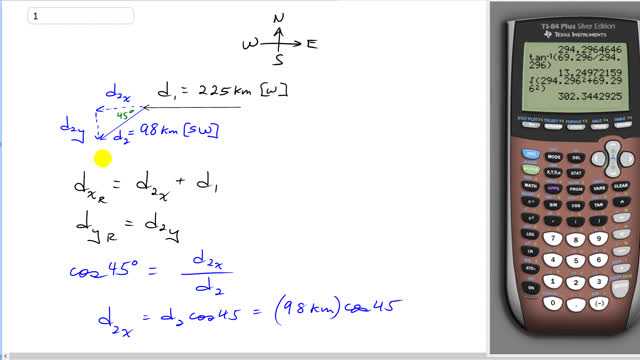
This is Giancoli Answers with Mr. Dychko. This car initially drives to the west— 225 kilometers— and then turns at a 45 degree angle because that's what south-west is and drives 98 kilometers. And the resultant displacement is gonna be from the origin where it started to where it finishes here so this in red line is d subscript R for resultant. And we are gonna figure out the components of the resultant and then based on those components, figure out all the details about the resultant. So we are gonna need to find this angle Θ in here its angle by which it's south of west and we'll need to find the length of this resultant and we'll use Pythagoras using the x-component of the resultant which will be d 1 plus the x-component of d 2 and then the y-component of the resultant is the y-component of d 2. So let's get started. So x-component of resultant is those two added together as I was just saying so this resultant is along this line here with length d 1 plus d 2 x and then the resultant is since d 1 has no component in the y-direction, the y-component of the resultant is entirely due to the y-component of d 2. Now we know cos of 45 degrees is adjacent over hypotenuse and so that's d 2 x over d 2 and multiply both sides by d 2 and then switch the sides around and then you get d 2 x is d 2 times cos 45 or 98 kilometers times cos 45 and make sure your calculator's in degree mode if you are using 45 degrees; if it's in radian mode, you will have to use π over 4 instead of 45 degrees. And then we get 69.296 kilometers and I should write that in here I guess. And for the y-component sin 45 is equal to cos 45 so I didn't put that in the calculator and you get the same answer, 69.296 kilometers. And the resultant in the x-direction then is this component of d 2 in the x-direction plus d 1—225 kilometers— and that gives 294.296 kilometers to the west is the x-component of the resultant. And its y-component is then the y-component of d 2. And define the angle of the resultant that's this angle in red Θ here the tangent of this Θ is the y-component divided by the x-component and so that's the y-component of the resultant— 69.296—divided by the x-component of the resultant— 294.296 kilometers—and then take the inverse tangent of that result and you get 13.25 degrees and that's the angle below the horizontal there. And then the length of the resultant is given by using Pythagoras and so we take the square root of the x-component of the resultant squared plus the y-component of the resultant squared and we get 302. And so our final answer then is 302 kilometers for the resultant displacement 13 degrees south of west and I'll explain south of west a little bit. So if this angle was zero, the thing would be going entirely west but it's deflected downwards slightly, it's deflected towards the west or sorry, let me say that again, it's deflected towards the south compared to west. So when you say south of west, you are saying to the south with respect to west or you know, to the south of west. Hopefully that kind of makes sense so there we go.