VIDEO TRANSCRIPT

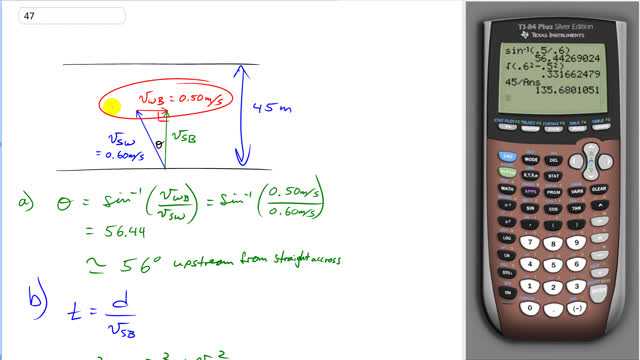
This is Giancoli Answers with Mr. Dychko. The velocity of the swimmer with respect to the water has to point somewhat upstream in order to compensate for the velocity of the water with respect to the bank which is 0.5 meters per second downstream. And the two velocities together will make the velocity of the swimmer with respect to the bank and this will be straight across and we'll have to figure out what this angle is in order to make that happen. So Θ would be the inverse sign then of the opposite divided by the hypotenuse this is a right triangle and so that's inverse sin of 0.5 meters per second opposite leg divided by 0.6 meters per second the hypotenuse and that gives about 56 degrees upstream compared to straight across. And the amount of time it will take is this distance 45 meters divided by the resulting velocity of the swimmer with respect to the river bank. Well, this is a right triangle again so we can use Pythagoras to figure v sb out and v sw hypotenuse squared equals v sb squared plus v wb squared. And then we'll solve for v sb by subtracting v wb from both sides and velocity of the swimmer with respect to the bank with a square root then of 0.6 meters per second squared minus 0.5 meters per second squared which is 0.3317. So we have the 45 meters divided by 0.3317 meters per second which gives about 140 seconds with two significant figures for her to cross the river bank to the other side.