
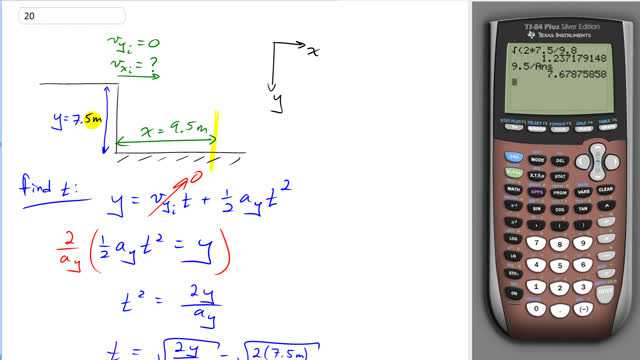
A ball is thrown horizontally from the roof of a building 7.5 m tall and lands 9.5 m from the base. What was the ball's initial speed?
In order to watch this solution you need to have a subscription.
This is Giancoli Answers with Mr. Dychko. This ball is thrown horizontally from the roof of a building and it falls 9.5 meters from the base of the building when it hits the ground and the building is 7.5 meters high. We'll take down to be the positive direction so that we don't need that pesky negative sign in our displacement downwards in our acceleration due to gravity and we'll take to the right being positive as usual. We'll start by first figuring out how long the ball spends in the air which depends only on the height of the building. We are told that the ball initially has no vertical velocity so it's not thrown up or down, it's thrown just horizontally but whatever this horizontal speed is, it's irrelevant for the amount of time the ball spends in the air; the time it spends in the air only depends on how far it falls. Okay. And this is zero so let's get rid of that we have this equation for displacement given this constant acceleration due to gravity downwards and we'll solve it for time because we already know what the height is and after we get rid of that term, we are left with this blue expression and then we multiply both sides by 2 over a y to get rid of the a and the 2 on the left side and then it ends up over on the right and then we have t squared, well, I take the square root of both sides there and then you are left with t equals square root of 2 times the vertical drop divided by the acceleration due to gravity. So that's square root of 2 times 7.5 divided by 9.8 and that gives 1.2372 seconds. Then we'll find the horizontal speed that it was launched with knowing how long it took to fall— which we just figured out— and knowing how far it went because we are told it goes 9.5 meters. So here's the expression for constant velocity; this displacement will be the velocity times time and we'll divide both sides by the time to solve for v x then we'll switch the sides around as well to have v x on the left and we have v x equals the horizontal displacement divided by time. So that's 9.5 meters divided by that whole time that we figured out before and that gives 7.7 meters per second when you round to two significant figures.