The pendulum in a grandfather clock is made of brass and keeps perfect time at $17 ^\circ \textrm{C}$. How much time is gained or lost in a year if the clock is kept at $29 ^\circ \textrm{C}$? (Assume the frequency dependence on length for a simple pendulum applies.) [Hint: See Chapter 8.]

VIDEO TRANSCRIPT

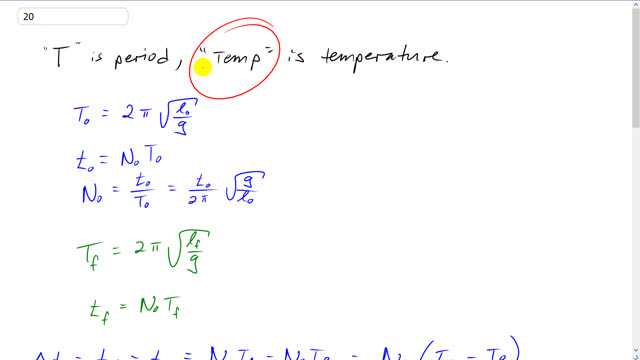
This is Giancoli Answers with Mr. Dychko. We're going to use the variable capital T to represent period of the pendulum in the grandfather clock. And we'll use the 4 letters, t-e-m-p, temp for the temperature because it's confusing to have the capital letter T stand for temperature on the one hand and then period on the other. So, to make things less confusing we'll say this t is period. So, when the grandfather clock is at 17 degrees, I believe it is, yeah, it keeps perfect time. And we'll call that period that the pendulum is swinging at t naught when it's at 17 degrees. And that period is going to be 2π times the square root of the length of the pendulum which we'll call l naught over g. And this is the formula for the period of a pendulum in terms of its length. And the amount of time that passes after the pendulum swings this many times back and forth is going to be the number of swings multiplied by the time for each swing. So, this is the period, the amount of time that passes per swing, maybe 15 seconds or something per swing. And this is the number of swings. And then this is the amount of time it genuinely passes in total. And we're interested in this because the way the clock works is that with all of its gears and so on, when the pendulum swings, it advances the minute hand a certain number of degrees so, it indicates a full day has passed after a certain number of swings. And, well, you know, when the grandfather clock indicates that a day has passed, it may or may not be true that a 24 hours has actually passed. It depends on how whether or not the clock is calibrated properly. But either way, it'll indicate a day has passed after this given number of swings, no matter what, because that's how the gearing ratio works. So, it'll pass the minute hand through 360 degrees 20 or 12 times, well, 24 times I guess, in a full day. And it'll take this many swings to indicate a full day. Now, when things are calibrated properly, it means that we'll have this much time genuinely passing divided by this amount of time for swing. And when we have this... This is the amount of time in a full year. And this is the period when it is properly calibrated and so that means this is the number of swings to indicate that a full year has passed. It's the number of swings that happens in a full year when everything is calibrated properly because we have the proper period here, we're told, it's the proper period when we have this particular length. And this is the amount of time in a year, t naught. OK. And then when the temperature increases to 29 degrees Celsius the length will change because there's going to be thermal expansion of the pendulum, it's going to be longer now and it's going to have a new period. And... And after this many swings, N naught, the grandfather clock will indicate that a year has passed but the amount of time that's actually passed will not be a year because the clock is no longer calibrated properly. But here's the amount of time that genuinely does pass. Here's the, this multiplying, N naught by the final period means that the clock will have indicated that a year has passed. And to figure out how much time is genuinely passed, we take this number of swings multiplied by the new period, Tf for final f final after the temperature's changed. And this is the amount of time that is genuinely passed. And our question is, how much is the clock off? And that's going to be this. What is the difference in time when the clock has indicated that a year has passed in the final case when its period is capital Tf minus the amount of time that that has actually passed when it's properly calibrated. So, t naught is the amount of number of seconds in a year and tf is the number of seconds that passes when you have this new period. So, we'll do substitutions now. So, this time, the tf, the total amount of time that passes after the clock has indicated that a year has passed, even though year has not actually passed, there's going to be N naught times the final period. And then we're gonna minus from that N naught times the period when it's properly calibrated. And we can factor out the N naught there. And then we'll substitute some things in. So, N naught is t naught over 2π times square root of g over l naught. And then the final period is 2π times the final length over g minus the period when it's properly calibrated which is 2π times square root l naught over g. And a bunch of things can factor out, the 2π can factor out in the numerator and then it cancels with the one in the denominator. So, it doesn't appear in this next line. And the square root g in the denominator can factor out from these two terms in the brackets and then that cancels with the square root g in the numerator here. And so that cancels. So, we're left with t naught over square root l naught times square root lf minus squared l naught. Then we turn our attention to this thermal expansion business. So, the final length after the temperature increases is going to be the initial length, l naught, plus the change in length. And the change in length is the coefficient of linear expansion times the original length times the change in temperature. And we can factor out l naught and get l naught times 1 plus α times change in temperature. So, we substitute that in for lf here. There. And square root l naught now becomes a common factor between these two terms inside the bracket and we can factor it out. And then it cancels with the square root l naught on the bottom out here. And so we have just t naught times square root 1 plus α times change in temperature minus 1. And then we can plug in numbers and get our answer. So, the amount of time that's passed is the amount of time that passes when it's properly calibrated, t naught, is 1 year. So, that's 365 and a quarter days year times 24 hours per day times 3600 a seconds per hour. And this gives us the number of seconds per year. And it times up by square root of 1 plus the coefficient linear expansion for brass 19 times 10 to the minus 6 times change in temperature of 29 degrees Celsius minus 17 degrees Celsius and then minus 1. And that gives us this many seconds and times by 1 minute for every 60 seconds. And it's about 60 minutes. So, this is the amount of time by which the clock is off. So, it's off by 60 minutes. And that's positive. So, that means that... Let me think about that for a second. So, that means tf is greater than t naught. And so the amount of time that's actually passed when the length has increased after indicate a year is greater than a year. So, this clock is running slow, 60 minutes slow.