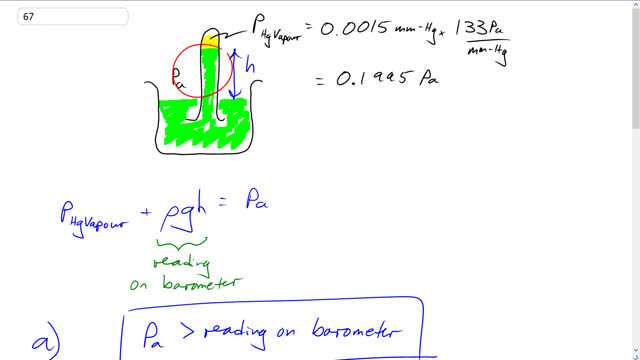
When using a mercury barometer (Section 10–6), the vapor pressure of mercury is usually assumed to be zero. At room temperature mercury’s vapor pressure is about 0.0015 mm-Hg. At sea level, the height $h$ of mercury in a barometer is about 760 mm.

VIDEO TRANSCRIPT

This is Giancoli Answers with Mr. Dychko. Here's a mercury barometer where this test tube is full of mercury and it's put upside down into this beaker and some column of this mercury will be supported within the test tube, and due to this air pressure pushing down. And we assume that there's no pressure in this space here. However, it turns out that there is some saturated vapor pressure for mercury at room temperature and it's really small, though, and it's 0.0015 millimeters of mercury which he can convert into pascals by multiplying by 133 pascals per millimeter of mercury. And that's 0.1995 pascals. So, the balance between pressures here is actually air pressure, and on the one hand pushing this column upwards. And then there's pressure due to the liquid column of density of mercury times g times the height of the column plus this vapor pressure which is in this space here. And, so this means that if you took the reading on the barometer and said that that's the pressure, the air pressure outside, that's going to be smaller than what it should be. The actual air pressure is the reading plus this very tiny saturated vapor pressure of mercury. So, the air pressure is greater than the reading on the barometer. The percent error is going to be the reading on the barometer minus the actual atmospheric pressure divided by the atmospheric pressure. And so that's ρ g h minus... Minus this from up here for the atmospheric pressure, and then divided by the true pressure. And these ρ g h's cancel and we're left with negative saturated vapor pressure of mercury divided by the actual pressure which is vapor pressure of mercury plus the pressured due to the column of mercury, liquid column. So, that's negative 0.15995 pascals divided by 0.1995 pascals plus 13.6 times 10 to the 3 kilograms per cubic meter, density of mercury, times 9.81 newtons per kilogram, gravitational field strength, times 760 millimeters which we write as times 10 to the minus 3 meters. And this gives a very small number of negative 2.0 times 10 to the minus 4 percent. So, I didn't write it here but there's also times by 100% here to turn this into a percent. So, it's a very negligible error, and it's good enough to just, you know, say that ρ d h is the atmospheric pressure because the difference is very small. However, if you were to use water, the percent error would be bigger, and the formula is the same. So, we have the saturated vapor pressure of water at standard temperature and pressure, so at 0 degrees Celsius, this is a saturated vapor pressure of water and we're using saturated vapor pressure because we assume that, you know, this device has been left on the table for a long, long, long time. So that water has had lots of time to evaporate and it will do so, up until the point where it saturates. So, we go negative 6.11 times 10 to the 2 pascals divided by atmospheric pressure of 1.013 times 10 to the 5 pascals at STP, times 100%. And you get 0.603% error, negative, if you neglect this vapor pressure in the top of the test tube.