Compare the value for the density of water vapor at exactly $100 ^\circ \textrm{C}$ and 1 atm (Table 10–1) with the value predicted from the ideal gas law. Why would you expect a difference?

VIDEO TRANSCRIPT

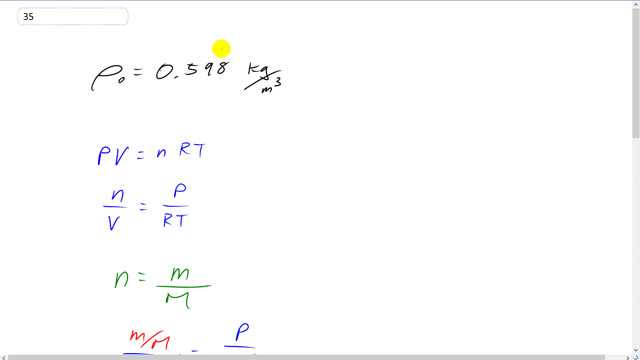
This is Giancoli Answers with Mr. Dychko. The density of steam according to table 10-1 is 0.598 kilograms per cubic meter. So, at 100 degrees Celsius ,that's the density of you know, you could say water but water vapor or steam. So, we're going to calculate what the theoretical density should be and compare that with what it actually is. This is what it actually is. And we have pressure times volume equals number of moles times universal gas constant times the temperature. And if we take number of moles divided by V, we can substitute the mass of the water divided by its molar mass for a number of moles. And that means we have mass divided by molar mass all over V equals pressure time over R T. So, this I divided by a bunch of things, I divided both sides by R T and P. And on the right hand side we end up with, R T and V I should say. On the right hand side we end up with n over V because the R T's cancel. On the left side you end up with P over R T since the V's cancel. And then switch the sides around. So, we're trying to make an equation for density. And we've got 1 step further by substituting for the number of moles as mass divided by molar mass and then multiply both sides by molar mass. And you get mass over volume equals pressure times molar mass over R T. And mass over volume is density. So, we can say density is pressure times molar mass divided by R times temperature. So, it's atmospheric pressure. So, we have 1.013 times 10 to the 5 pascals times the molar mass and you look in the periodic table of elements in the back cover of the text book and you'll see the molar mass in grams per mole so, we take those numbers and times by 10 to the minus 3 to get kilograms per mole. So, it's 2 hydrogens, so, that's 2 times 1.00794 grams per mole plus 15.9994 grams per mole for oxygen and then all that's divided by 8.314 joules per mole kelvin and then times by the temperature in kelvin, 100 degrees Celsius plus 273.15. And that gives 0.58807 kilograms per cubic meter is the theoretical density for water if it was an ideal gas. And we can see the difference between the actual density and the theoretical density. The actual being 0.598 kilograms per cubic meter, and the theoretical being 0.588 kilograms per cubic meter for a difference of 0.02. The actual density is 0.02 kilograms per cubic meter greater than the theoretical density. And so the real density is higher than predicted and that's to be expected because there is a polar attraction between the water molecules since they, you know, 2 hydrogens and an oxygen, and the oxygen has a high electronegativity, so it attracts the a electron and holds on to it more tightly than the hydrogen does. And so the water molecule effectively is a sort of a positiveness at this end where the hydrogens are, and a negativeness where the oxygen is. And then that attracts the next water molecule nearby which will have positive here and negative there and opposites attract. And so this water molecule is polar because it has a charge distribution. So, there's attraction between the water molecules which would increase the density. And so the real density is higher than predicted because this prediction assumes that there's no such polar attraction. An ideal gas has only elastic collisions as interactions between the molecules. Whereas in water in fact it has also these polar attraction interactions. And also the ideal gas law doesn't work very well when the temperature is near a phase transition and that's the case here, 100 degrees Celsius is the temperature when you transition between liquid and gas. So, those are two good reasons why the predicted density should not be the same as the real density.