Show that the rms speed of molecules in a gas is given by $v_{rms} = \sqrt{\dfrac{3P}{\rho}}$, where $P$ is the pressure in the gas and $\rho$ is the gas density.

VIDEO TRANSCRIPT

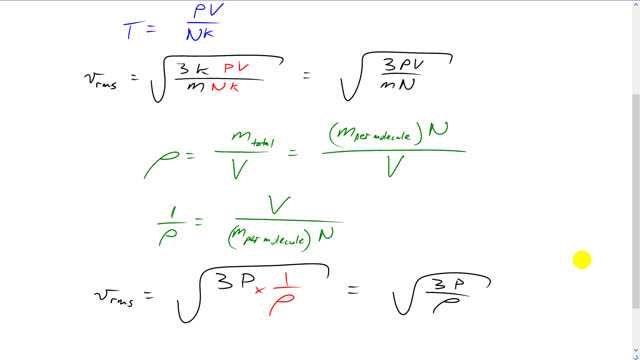
This is Giancoli Answers with Mr. Dychko. Rms speed of a molecule is square root of 3 times Boltzmann's constant times temperature over m. Now, we need to replace some things in here in order to make it look like this over here. So, there's a pressure factor in there. So, let's return to our ideal gas law. And we're going to replace temperature with this stuff containing pressure and that'll get us 1 step of the way. So, P V equals n K T. I chose this form for the ideal gas law because it'll also cancel away our K, the Boltzmann's constant. I could have chosen n R T but then that would make things more confusing because we'd have moles and we'd have this universal gas constant, R, and that's no good. So, we'll choose T is P V over the number of molecules times K. After dividing both sides by N K you get this. And then substitute that in for T. So, T is now P V over N K. And the K's cancel, leaving us with V rms is square root of 3 times pressure times volume over the mass of a single molecule times the number of molecules. Now, let's turn to density. Density is the total mass divided by the volume. And the total mass is the mass per molecule which is what this little m is here, mass for a single molecule, multiplied by the number of molecules. This gives total mass here over a volume. And taking the reciprocal of both sides, makes it easier to substitute into here. And we have 1 over the density is, flip this fraction over, V over mass per molecule times N. And that's what this is, V over mass per molecule times N. And so we substitute 1 over density in place of V over m N. And this gives us what we want, V over mass is square root of 3 times pressure divided by density.