
How many revolutions per minute would a 25-m-diameter Ferris wheel need to make for the passengers to feel “weightless” at the topmost point?
In order to watch this solution you need to have a subscription.
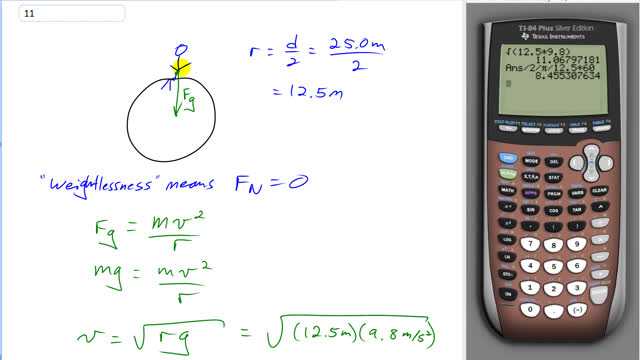
This is Giancoli Answers with Mr. Dychko. The sensation of weightlessness means that you are not experiencing any normal force; it's the sensation when the ground is not pushing back up on you and it's the sensation of free falling and that will only happen when you have a free-body diagram where there's only gravity pulling down on the person, there's no normal force going up. The person will be in free fall, for a moment, at the top of the ferris wheel. And so it's a little bit of bookkeeping work; we have to get the radius of this ferris wheel and it's half the diameter so that's 25 meters over 2 and that's 12.5 meters. So, the net force on this person is just the one force, the force of gravity and that equals mass times acceleration but we can tell that the acceleration is centripetal acceleration, it's radial acceleration. And that makes it v squared over r. So, this is is Newton's second law, where I have written ma but instead of a, I have written v squared over r, in place of a. And force of gravity is mg and then we can say, mg equals m v squared over r. Divide both sides by m, multiply both sides by r and you get that the v squared is rg and then take the square root of both sides and so v is the square root of the radius times acceleration due to gravity. So we have square root of 12.5 meters times 9.8 meters per second squared, which is 11.068 meters per second. So that's the speed that the first wheel will have to have for a point on the rim of the ferris wheel but what we are told to find is the rpm, revolutions per minute. So we'll take this speed, multiply it by one full revolution for every circumference of the ferris wheel, which is 2πr, 2 times π times the radius, 12.5 meters. And then times by 60 seconds for every minute and the seconds will cancel and the meters will cancel leaving us with revolutions per minute. So that's 8.5 revolutions per minute.
Why is the Fg not negative? The vector is pointing downwards.
Nevermind. I see it. It is bc the centripetal acceleration is negative so we can divide both sides by -1 making it positive.