Planet A and planet B are in circular orbits around a distant star. Planet A is 7.0 times farther from the star than is planet B. What is the ratio of their speeds $\dfrac{v_A}{v_B}$?

VIDEO TRANSCRIPT

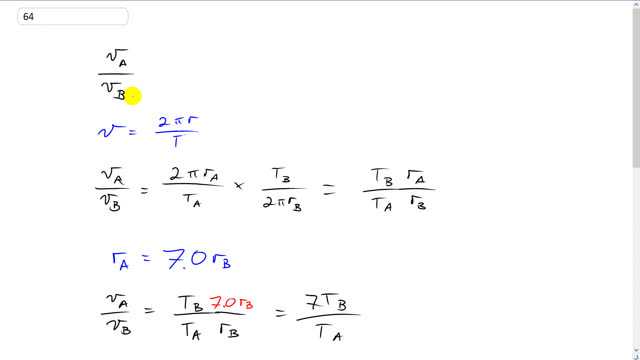
This is Giancoli Answers with Mr. Dychko. Our job is to the find the ratio of the speeds of these two planets as they orbit around the same star. So and that's a note to keep in mind that I said "same star" and the, when you are comparing two things that are orbiting, these Kepler's laws only work if they are orbiting the same thing. So, all the planets have to orbit the same star for example, or all the moons have to orbit the same planet. OK So, speed of anything going in a circle is the circumference of the circle, 2πr, divided by the period, or the time it takes to do one full circle. So, I'm gonna make replacements here for v A is 2π r A divided by T A. And then divide by v B is the same as multiplying by the reciprocal of its speed so I'm gonna multiply by T B over 2πr B. So instead of having a fraction divided by another fraction, I'm just multiplying by the reciprocal of the bottom thing. And then the 2π's cancel and we are left with T B r A divided by T A r B. That's the ratio of the speeds. Now, the distance to planet A is 7 times the distance to planet B so we'll make a substitution for r A, and we'll call it 7 times r B and then the r B's cancel leaving us with 7T B over T A. And this is just begging for Kepler's law because we know that the ratio of the period squared equals the ratio of the orbital radii cubed; that's Kepler's law. And so, if we take the square root of both sides here, we get the ratio T B over T A which we want to know so we can make a substitution here. And we have this ratio in terms of the radii, which we know because we know that r A is 7 times r B. So, we'll make that substitution here. And they have this exponent 3 over 2 because when you have a square root, you can think of a square root as the same as exponent one-half. And so, we have a cube there already so 3 times a half is 3 over 2. So that gives us this exponent. And r B over 7r B is gonna be 1 over 7. So we have the ratio of the periods, T B over T A, is one-seventh to the power of 3 over 2. So then when we return back to this formula here for the ratio of the speeds, and we make a substitution for the ratio of periods, T B over T A, and call it one-seventh of 3 over 2. And so we have 7 divided by 7 to the 3 over 2 is the same as multiplying by one-seventh of 3 over 2. And so that's 7 to the power of 1 minus 3 over 2, which is 7 to the power of negative of a half. So the answer to our question is 7 to the power of negative and a half, which is 0.38 is the ratio of the speeds of these planet.