Calculate the speed of a satellite moving in a stable circular orbit about the Earth at a height of 4800 km.

VIDEO TRANSCRIPT

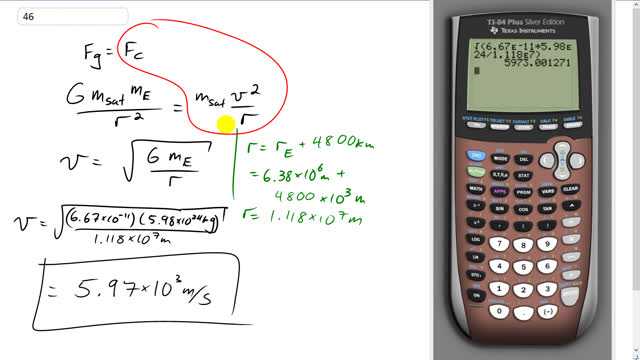
This is Giancoli Answers with Mr. Dychko. At constant speed, the centripetal force is the net force; so it's mass times acceleration and acceleration, when you are going in a circle, is v squared over r, when v is constant. And gravity is what's providing the centripetal force so that means G mass of the satellite times mass of the Earth divided by r squared is gonna be equal to centripetal force. And r is the distance from the center of the Earth to the satellite and so, we are told that the satellite is 4800 kilometers above the Earth, above the surface of the Earth, so we have to add that to the radius of the Earth to get the total distance from the center of the Earth to the satellite. And we are gonna multiply both sides by r here to cancel one of the r's and get rid of it there; divide both sides by the mass of the satellite, take the square root of both sides and we get the speed required is gonna be square root of universal gravitational constant times mass of the Earth divided by r, and that's r to the power of 1. So that's square root of 6.67 times 10 to the minus 11 times 5.98 times 10 to the 24 kilograms— mass of the Earth— divided by 1.118 times 10 to the 7 meters— distance from the center of the Earth to the satellite— and that's 5.97 times 10 to the 3 meters per second should be the speed of the satellite to make sure it stays in orbit.