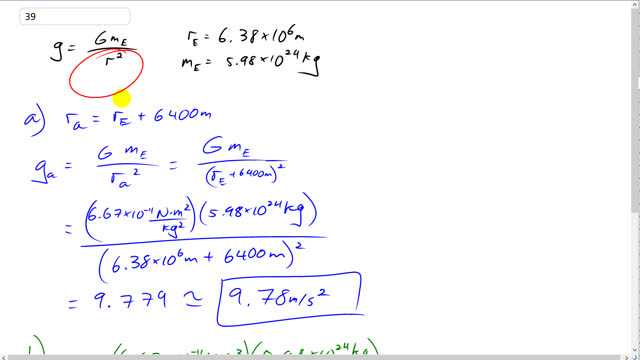
Calculate the effective value of g, the acceleration of gravity, at

VIDEO TRANSCRIPT

This is Giancoli Answers with Mr. Dychko. Acceleration due to gravity is universal gravitational constant times the mass of the planet, Earth in this case, divided by the distance to the position you are interested in. Knowing the acceleration of gravity at, to the center of the mass squared, and the distance in part (a) will be either radius of the Earth plus the 6400 meters above the surface. And so we have, in the case (a), the acceleration due to gravity is capital G times m E divided by radius of the Earth plus 6400. So that's 6.67 times 10 to the 11 newton meters squared per kilogram squared times 5.98 times 10 to the 24 kilograms— mass of the Earth— divided by 6.38 times 10 to the 6 meters plus 6400 meters and we square that sum on the bottom, and we get 9.78 meters per second squared. So it hasn't changed by much, 9.80 at the surface, so even when you go almost six and a half kilometers above the surface, acceleration due to to gravity doesn't change very much. But then when you go, 6400 kilometers, and I substituted, times 10 to the 3 meters here, always keeping the units in mks units— meters, kilograms, seconds— and we see, in this case, same numbers except you are going radius of the Earth plus 6400 times 10 to the 3 meters, we get 2.44 meters per second squared, acceleration due to gravity, 6400 kilometers above the surface of the Earth.