Determine the distance from the Earth’s center to a point outside the Earth where the gravitational acceleration due to the Earth is $\dfrac{1}{10}$ of its value at the Earth’s surface.

VIDEO TRANSCRIPT

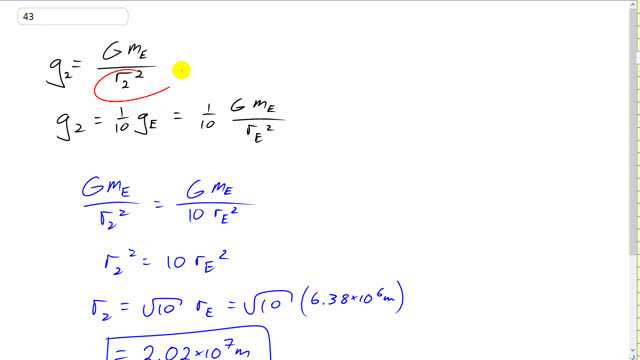
This is Giancoli Answers with Mr. Dychko. The acceleration due to gravity, at some point, above the Earth's surface is gonna be G times mass of the Earth divided by the distance to the Earth's center squared at this position. And then we are told that g 2 is one-tenth of the acceleration due to gravity at the surface. At the surface, acceleration due to gravity is G mass of the Earth divided by the radius of the Earth squared. And so we substitute this in for g 2, and we get this, G m E over r 2 squared equals G m E divided by r E squared times a tenth. So we have divide by 10 down there. And the capital G and mass of the Earth factors, both cancel on both sides and then we can raise both sides to the negative exponent 1, or, in other words, take the reciprocal of both sides and we get r 2 squared equals 10 times r E squared, and we need to take the square root of both sides to figure out what this r 2 is; we end up with square root 10 times r E. So that's square root 10 times 6.38 times 10 to the 6 meters, which gives 2.02 times 10 to the 7 meters, is the distance from the center of the Earth to this position, where the acceleration due to Earth is one-tenth what it is on the surface.