
What is the magnitude of the acceleration of a speck of clay on the edge of a potter’s wheel turning at 45 rpm (revolutions per minute) if the wheel’s diameter is 35 cm?
In order to watch this solution you need to have a subscription.
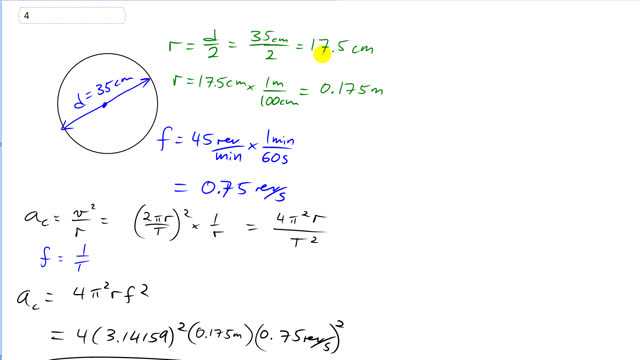
This is Giancoli Answers with Mr. Dychko. Let's begin the solution by dividing this diameter by 2 to get the radius since the radius is what we are gonna use in our centripetal acceleration formulas. So 35 centimeters divided by two 17.5 centimeters and then we'll convert that into meters because we always want meters, kilograms, seconds, those types of units, mks units, for our formulas. So we have 17.5 centimeters times 1 meter for every 100 centimeters; centimeters cancel giving us 0.175 meters. The frequency is 45 revolutions per minute but we wanna turn that into revolutions per second; again we want, meters, kilograms and seconds, mks units, and so let's get rid of the minutes there. So we times by 1 minute for every 60 seconds and we have 0.75 revolutions per second. Now, centripetal acceleration is the tangental velocity, or the speed squared divided by the radius of the curve that it's going around. And the speed v is 2πr over T—that's the circumference on top—2πr divided by the period on the bottom. I'm driving this handy formula down here that we can directly plug our numbers into. So, I have rewritten this as v, 2πr over period squared and then divided by r is the same as times by 1 over r because this could be a little bit confusing to have fractions within fractions so I am writing them side by side like this. And squaring this bracket gives us 4 and then pi squared over T squared and the r would be squared except that there's one r here which cancels with one of the r's there leaving us with just a single r. Now, frequency is the reciprocal of period and we are given frequency so let's substitute that in for this period here so you know, frequency squared then is gonna be 1 over period squared and this whole thing is the same as 4π squared r times 1 over period squared and 1 over period squared is frequency squared so we can write this line here. This is a handy way to write centripetal acceleration; it always works whenever you are given frequency and radius. And so we have 4 times pi squared times 0.175 meters times the 0.75 revolutions per second squared and we get 3.9 meters per second squared is the centripetal acceleration.
Why f^2=1/t^2 is because the periods is squared
Hi joeotilio25, to explain why , first you need to notice that . Period and frequency are reciprocals of each other, which you can also tell by their units. The units of frequency are "Hz", but "Hz" means "cycles / second". The word cycles is not really a unit, so Hz is essentially "1 / seconds", which is the reciprocal of period which has units of seconds. So, since , when you square both sides you get . Squaring both sides was useful for substituting into the centripetal acceleration formula, as shown in the video.
All the best,
Mr. Dychko
Where did revolutions go? I get the answer as 0.39 m-rev/s2.
Hi bbailey1956, that's a good question about where the "revolutions" unit goes. It turns out that "revolutions" is not actually a unit. The wikipedia article on the topic calls "revolutions" a semantic annotation (see https://en.wikipedia.org/wiki/Revolutions_per_minute), which is a fancy way of saying "a word that gives meaning to something else being measured". A good way to look at it is that revolutions are not measured, but rather, the time of a revolution is measured. Only things that are measured (or measurable) get units. The length of a revolution is measurable, and so is the time, but not the revolution itself. To call a revolution a unit would be like measuring the width of a piece of paper and calling "paper" a unit. "Paper" is just a word telling you what was measured.
I can totally see the confusion here, since it's just a habit on this topic of "rpm" to include "rev" among the units in a calculation, even though it is in fact not a unit.
@bbailey1956, I almost forgot to comment on your final answer. I've checked the work, and the answer appears correct, and I can't think of where the mistake would be in your work to have an answer different by a factor of ten, so you'll just have to inspect your work carefully.
All the best,
Mr. Dychko