
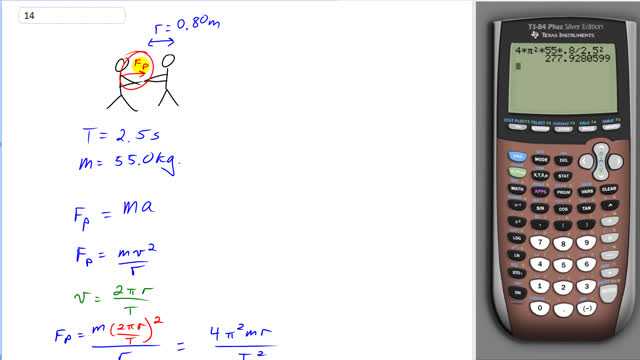
On an ice rink two skaters of equal mass grab hands and spin in a mutual circle once every 2.5 s. If we assume their arms are each 0.80 m long and their individual masses are 55.0 kg, how hard are they pulling on one another?
In order to watch this solution you need to have a subscription.
This is Giancoli Answers with Mr. Dychko. As the skaters go around in a circle, they are pulling on each other and applying a force towards the center of the circle, so that's, F P for pole. And they go once around in a circle in two and a half seconds; each of them has a mass of 55 kilograms and they are traveling in a circle of radius, 0.8 meters. So the pulling force is the only force— let's consider the left-hand person— it's the only force on the left-hand person and it's equal to their mass times acceleration, that's Newton's second law. Acceleration, in this case, is centripetal acceleration so it's v squared over r; and we can calculate the speed by going 1 full circumference, 2πr, divided by the period or the time it takes to go that full circumference; and we'll substitute that in for v. And so we have, pulling force is m v squared over r but the v is now 2πr over T, capital T for period, and that makes 4 π squared times m times a single r because r squared divided by r makes r to the power of 1 and all divided by period squared, T squared. So that's 4 π squared times 55 kilograms times 0.8 meters divided by two and a half second squared which is 280 newtons, with two significant figures.
Wow