
A nylon string on a tennis racket is under a tension of 275 N. If its diameter is 1.00 mm, by how much is it lengthened from its untensioned length of 30.0 cm?
In order to watch this solution you need to have a subscription.
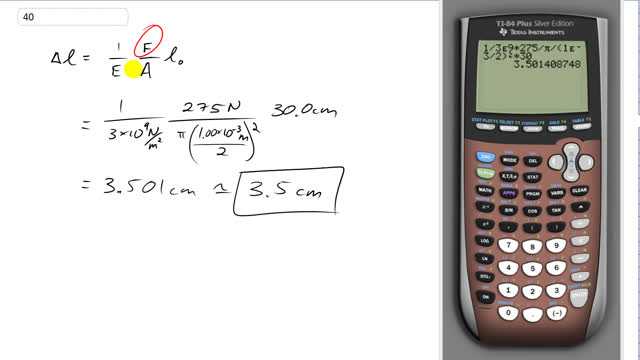
This is Giancoli Answers with Mr. Dychko. The change in length of the nylon string will be 1 over the elastic modulus, also called the Young's Modulus, times the force applied on the string divided by its cross-sectional area times its original length. So cross-sectional area is πr squared, r is the radius of the nylon string, and so we are given 1 millimeter which is 1.00 times 10 to the minus 3 meters for the diameter so we have to divide that by 2 and then square that and times by π to get the cross-sectional area of the nylon string. And Young's Modulus for nylon is 3 times 10 to the 9 newtons per meter squared times by 275 newtons—force that is stretching the nylon string— times its original length of 30.0 centimeters and because I have put in centimeters here, our answer's gonna be in centimeters as well and it should be about 3.5 centimeters of stretching.