At depths of 2000 m in the sea, the pressure is about 200 times atmospheric pressure $(1 \textrm{ atm} = 1.0 \times 10^5 \textrm{ N/m}^2)$. By what percentage does the interior space of an iron bathysphere’s volume change at this depth?

VIDEO TRANSCRIPT

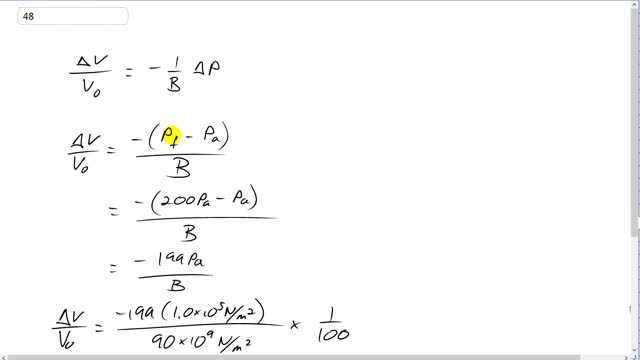
This is Giancoli Answers with Mr. Dychko. We know the fractional change in volume will equal the negative of 1 over the bulk modulus of the iron times its change in pressure that it experiences. So the final pressure is at the bottom of the ocean: 200 times atmospheric pressure and we assume that it starts at atmospheric pressure. So that's 200Pa minus Pa which is 199Pa. So fractional change in volume is negative 199 times 1.0 times 10 to the 5 newtons per square meter— that's atmospheric pressure— divided by the bulk modulus of iron, 90 times 10 to the 9 newtons per square meter and times by 1 over 100 to turn it into a percent and that gives negative 2 times 10 to the negative 6 percent and the negative sign means the volume is gonna decrease by this much.