How much pressure is needed to compress the volume of an iron block by 0.10%? Express your answer in $\textrm{N/m}^2$, and compare it to atmospheric pressure $(1.0 \times 10^5 \textrm{ N/m}^2)$.

VIDEO TRANSCRIPT

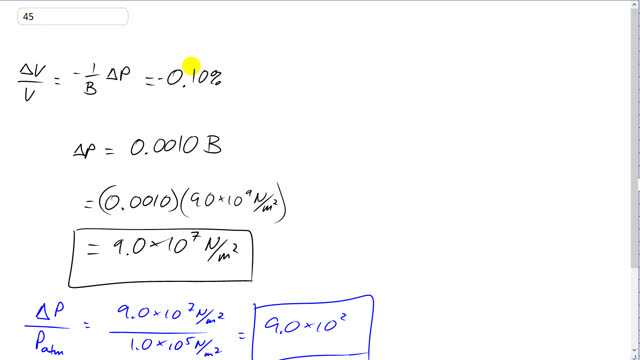
This is Giancoli Answers with Mr. Dychko. The fractional change in volume is the negative of 1 over the bulk modulus of the material times the change in pressure applied to it and we are told that the iron block will be compressed by 0.1 percent so compression means this ΔV is gonna be negative so we'll make this negative 0.1 percent. And multiply both sides of this by B and you know cancel the negative's we have the additional pressured required to compress this block is gonna be 0.0010 times B. So that's 0.0010 times bulk modulus of iron of 90 times 10 to the 9 newtons per square meter which is 9.0 times 10 to the 7 newtons per square meter. And comparing that to atmospheric pressure, we take this additional pressure needed to squish the iron block divided by 1 times 10 to the 5 newtons per square meter and this additional pressure is gonna be 900 times atmospheric pressure, 9.0 times 10 to the 2.